List of formulae

Mensuration

Area and Perimeter of Plane Figures

Name	Shape	Perimeter	Area
Triangle		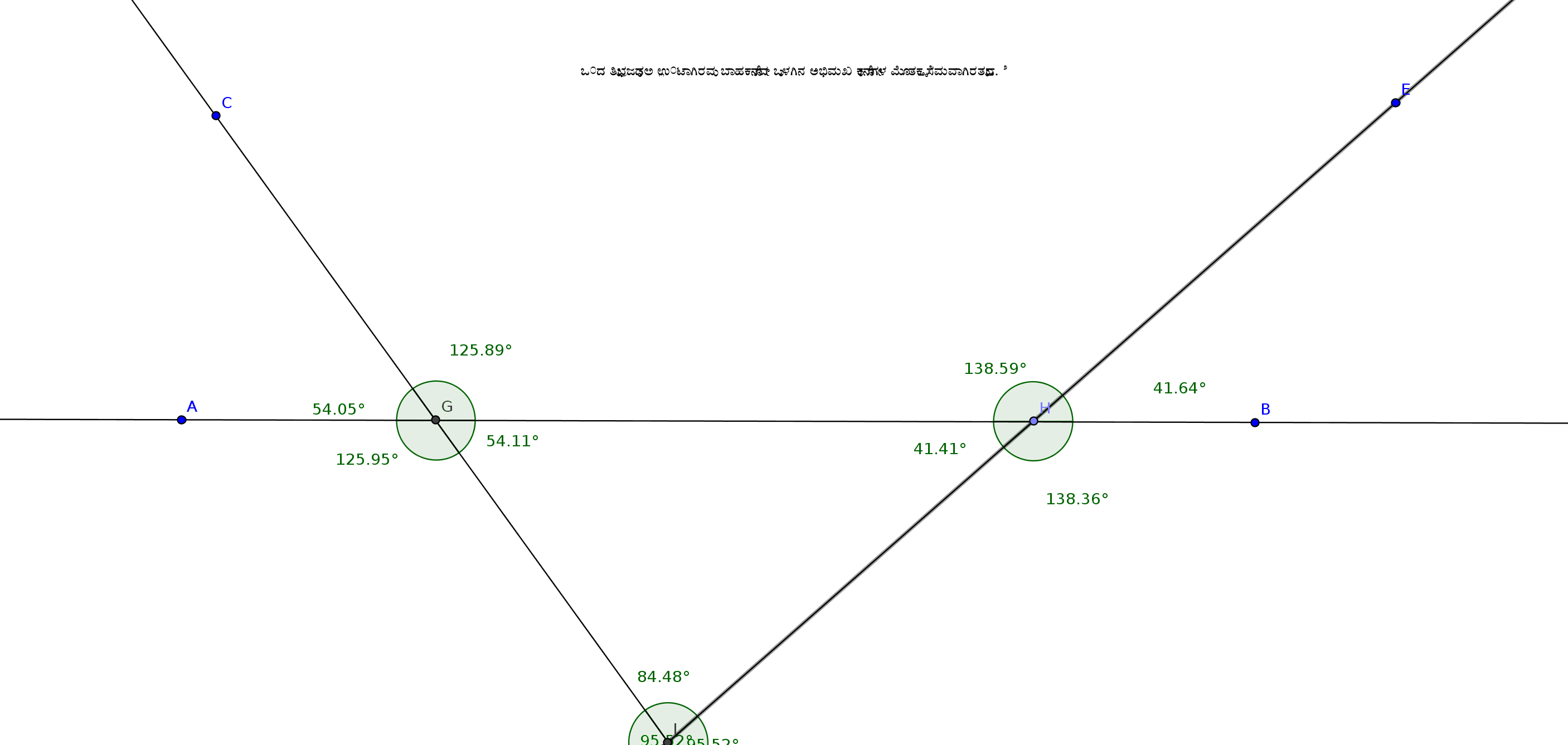(a+b+c), where a, b, c are sides	${\frac {1}{2}}bh$ where "h" is the height from any vertex to the opposite side "b"
Circle	$2*{\pi }*r$	${\pi }*r^{2}$
type square root		${\sqrt {25}}$

LSA(CSA) TSA & VOLUME of Solid Figures

Name of the Solid	LSA(CSA)in sq.units	TSA in sq.units	VOLUME in cubic units
CUBE	$4l^{2}$ Where lenght(l)=breadth(b)=height(h)	$6l^{2}$	$l^{3}$
CUBOID	$2h(l+b)$	$2(lb+bh+lh)$	$lbh$
PRISM 1)EQUILATERAL TRIANGLE RIGHT PRISM	$Ph$ Where P=3a is the perimeter of base triangle	$2B+Ph$ Where B=${\frac {{\sqrt {3}}a^{2}}{4}}$ is the area of base	$Bh$ Where B=${\frac {{\sqrt {3}}a^{2}}{4}}$
2)SQUARE BASED RIGHT PRISM	$Ph$ Where P=4a is the perimeter of base square	$2B+Ph$ Where B=$a^{2}$ is the area of base	$Bh$ Where B=$a^{2}$
PYRAMID 1)EQUILATERAL TRIANGLE BASED RIGHT PYRAMID	${\frac {1}{2}}Pl$ Where P=3a is the perimeter of base triangle l is the slant height	$B+{\frac {1}{2}}Pl$ Where B=${\frac {{\sqrt {3}}a^{2}}{4}}$ is the area of base	${\frac {1}{3}}Bh$ Where B=${\frac {{\sqrt {3}}a^{2}}{4}}$
2)SQUARE BASED RIGHT PYRAMID	${\frac {1}{2}}Pl$ Where P=4a is the perimeter of base square l is the slant height	$B+{\frac {1}{2}}Pl$ Where B=$a^{2}$ is the area of base	${\frac {1}{3}}Bh$ Where B=$a^{2}$
CYLINDER	$2{\pi }rh$ Where r is the radius of circular base	$2{\pi }r(r+h)$ where "h" is the height of cylinder	${\pi }r^{2}h$
CONE	${\pi }rl$ Where l is the slant height	${\pi }r(l+r)$ Where r is the radius of circular base	${\frac {1}{3}}{\pi }r^{2}h$ Where h is the height or depth of the cone
FRUSTUM OF CONE	${\pi }(r_{1}+r_{2})l$ Where l=${\sqrt {h^{2}+(r_{1}-r_{2})^{2}}}$	Failed to parse (syntax error): {\displaystyle π{{(r_{1}+r_{2})l+r_{1}^2+r_{2}^2}}} Where $r_{1}$ & $r_{2}$ are the radii of two bases$(r_{1}>r_{2})$	${\frac {1}{3}}{\pi }h(r_{1}^{2}+r_{2}^{2}+r_{1}r_{2})$ Where h is the height or depth of the frustum ofcone
SPHERE	$4{\pi }r^{2}$	$4{\pi }r^{2}$	${\frac {4}{3}}{\pi }r^{3}$
HEMISPHERE	$2{\pi }r^{2}$	$3{\pi }r^{2}$	${\frac {2}{3}}{\pi }r^{3}$