Difference between revisions of "Properties of cyclic quadrilateral"

From Karnataka Open Educational Resources
Jump to navigation Jump to search
(Created page with "{{subst:Math-Activity}}")
 
m (added Category:Circles using HotCat)
 
(4 intermediate revisions by 2 users not shown)
Line 1: Line 1:
−
__FORCETOC__
+
Relation between the angles of a cyclic quadrilateral are explored with this hand on activity.
−
=Activity - Name of Activity=
+
 
 +
===Objectives===
 +
*In a cyclic quadrilateral the sum of opposite interior angles is 180 degrees.
 +
*In a cyclic quadrilateral the exterior angle is equal to interior opposite angle
 +
===Estimated Time===
 +
45 minutes
 +
 
 +
===Prerequisites/Instructions, prior preparations, if any===
 +
* Circles and quadrilaterals should have been covered.
 +
 
 +
===Materials/ Resources needed===
 +
Non digital: Coloured paper, pair if scissors, sketch pen, carbon paper, geometry box
 +
 
 +
This activity has been taken from the website http://mykhmsmathclass.blogspot.in/2007/11/class-ix-activity-16.html
  
−
==Estimated Time==
+
[[File:c.q.jpeg|300px|link=http://karnatakaeducation.org.in/KOER/en/index.php/File:C.q.jpeg]]
  
−
==Materials/ Resources needed==  
+
===Process (How to do the activity)===
−
==Prerequisites/Instructions, if any==
+
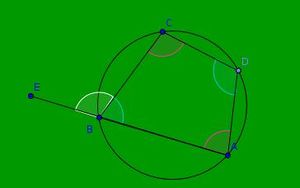
Note: Refer the above geogebra file to understand the below mentioned labelling.,br>
−
==Multimedia resources==
+
# Draw a circle of any radius on a coloured paper and cut it.
−
==Website interactives/ links/ simulations/ Geogebra Applets==
+
# Paste the circle cut out on a rectangular sheet of paper.
−
==Process (How to do the activity)==
+
# By paper folding get chords AB, BC, CD and DA in order.
−
==Developmental Questions (What discussion questions)==
+
# Draw AB, BC, CD & DA. A cyclic quadrilateral ABCD is obtained.
−
==Evaluation (Questions for assessment of the child)==
+
# Make a replica of cyclic quadrilateral ABCD using carbon paper.
−
==Question Corner==
+
# Cut the replica into 4 parts such that each part contains one angle .
−
==Activity Keywords==
+
# Draw a straight line on a paper.
 +
# Place angle BAD and angle BCD adjacent to each other on the straight line.Write the observation.
 +
# Place angle ABC and angle ADC adjacent to each other on the straight line . Write the observation.
 +
# Produce AB to form a ray AE such that exterior angle CBE is formed.
 +
# Make a replica of angle ADC and place it on angle CBE . Write the observation.
 +
* '''Developmental Questions (What discussion questions)'''
 +
# How do you take radius ?
 +
# What is the circumference ?
 +
# What is a chord ?
 +
# What is a quadrilateral ?
 +
# Where are all four vertices of a quadrilateral located ?
 +
# What part are we trying to cut and compare ?
 +
# What can you infer ?
 +
* '''Evaluation Questions''' 
 +
# Angle BAD and angle BCD, when placed adjacent to each other on a straight line, completely cover the straight angle.What does this mean ?
 +
# Angle ABC and angle ADC, when placed adjacent to each other on a straight line, completely cover the straight angle.What can you conclude ?
 +
# Compare angle ADC with angle CBE.
 +
# Name the two properties of cyclic quarilaterals.
 +
__FORCETOC__
  
−
'''To link back to the concept page'''
+
[[Category:Circles]]
−
<nowiki>
 
−
[http://karnatakaeducation.org.in/KOER/en/index.php/'''Give the link of the page name from where activity was given''' Back]
 

Latest revision as of 17:25, 5 November 2019

Relation between the angles of a cyclic quadrilateral are explored with this hand on activity.

Objectives

  • In a cyclic quadrilateral the sum of opposite interior angles is 180 degrees.
  • In a cyclic quadrilateral the exterior angle is equal to interior opposite angle

Estimated Time

45 minutes

Prerequisites/Instructions, prior preparations, if any

  • Circles and quadrilaterals should have been covered.

Materials/ Resources needed

Non digital: Coloured paper, pair if scissors, sketch pen, carbon paper, geometry box

This activity has been taken from the website http://mykhmsmathclass.blogspot.in/2007/11/class-ix-activity-16.html

C.q.jpeg

Process (How to do the activity)

Note: Refer the above geogebra file to understand the below mentioned labelling.,br>

  1. Draw a circle of any radius on a coloured paper and cut it.
  2. Paste the circle cut out on a rectangular sheet of paper.
  3. By paper folding get chords AB, BC, CD and DA in order.
  4. Draw AB, BC, CD & DA. A cyclic quadrilateral ABCD is obtained.
  5. Make a replica of cyclic quadrilateral ABCD using carbon paper.
  6. Cut the replica into 4 parts such that each part contains one angle .
  7. Draw a straight line on a paper.
  8. Place angle BAD and angle BCD adjacent to each other on the straight line.Write the observation.
  9. Place angle ABC and angle ADC adjacent to each other on the straight line . Write the observation.
  10. Produce AB to form a ray AE such that exterior angle CBE is formed.
  11. Make a replica of angle ADC and place it on angle CBE . Write the observation.
  • Developmental Questions (What discussion questions)
  1. How do you take radius ?
  2. What is the circumference ?
  3. What is a chord ?
  4. What is a quadrilateral ?
  5. Where are all four vertices of a quadrilateral located ?
  6. What part are we trying to cut and compare ?
  7. What can you infer ?
  • Evaluation Questions
  1. Angle BAD and angle BCD, when placed adjacent to each other on a straight line, completely cover the straight angle.What does this mean ?
  2. Angle ABC and angle ADC, when placed adjacent to each other on a straight line, completely cover the straight angle.What can you conclude ?
  3. Compare angle ADC with angle CBE.
  4. Name the two properties of cyclic quarilaterals.
Retrieved from "https://karnatakaeducation.org.in/KOER/en/index.php?title=Properties_of_cyclic_quadrilateral&oldid=32441"