Difference between revisions of "A Kite and its properties"
Jump to navigation
Jump to search
| Line 98: | Line 98: | ||
==Concept #2. Measurements in a kite== | ==Concept #2. Measurements in a kite== | ||
===Learning objectives=== | ===Learning objectives=== | ||
| + | # A kite has two pairs of congruent sides. | ||
| + | # Its diagnols intersect at right angles. | ||
| + | # The sum of its four sides would be its perimetre. | ||
| + | # Its area is given by the formula <math>1/2 x product of its diagnols </math> | ||
===Notes for teachers=== | ===Notes for teachers=== | ||
| − | ===Activity No # === | + | ===Activity No # Deriving formula for area of a kite=== |
{| style="height:10px; float:right; align:center;" | {| style="height:10px; float:right; align:center;" | ||
|<div style="width:150px;border:none; border-radius:10px;box-shadow: 5px 5px 5px #888888; background:#f5f5f5; vertical-align:top; text-align:center; padding:5px;"> | |<div style="width:150px;border:none; border-radius:10px;box-shadow: 5px 5px 5px #888888; background:#f5f5f5; vertical-align:top; text-align:center; padding:5px;"> | ||
''[http://www.karnatakaeducation.org.in/?q=node/305 Click to Comment]''</div> | ''[http://www.karnatakaeducation.org.in/?q=node/305 Click to Comment]''</div> | ||
|} | |} | ||
| − | *Estimated Time | + | *Estimated Time : 30 minutes. |
| − | *Materials/ Resources needed | + | *Materials/ Resources needed: Laptop, geogebra file, projector and a pointer. |
*Prerequisites/Instructions, if any | *Prerequisites/Instructions, if any | ||
| − | *Multimedia resources | + | # They should know a kite and its properties. |
| + | # The students should know the concept of an area. | ||
| + | # They should know the formula to find the area of a triangle. | ||
| + | *Multimedia resources: Laptop | ||
*Website interactives/ links/ / Geogebra Applets | *Website interactives/ links/ / Geogebra Applets | ||
| − | *Process | + | *Process: |
| − | *Evaluation | + | # The teacher can project the geogebra file on kite. |
| − | *Question Corner | + | # Show them that a kite is made of two isosceles traingles. |
| + | # Sum of areas of these two triangles would be the area of the kite. | ||
| + | *Developmental Questions | ||
| + | # What is a kite / | ||
| + | # What are the properties of a kite. | ||
| + | # What other figures can you see in a kite ? | ||
| + | # What types of triangles are these ? | ||
| + | # Identify the two isosceles triangles ? | ||
| + | # What is the formula to find the area of a triangle ? | ||
| + | *Evaluation: | ||
| + | # Choosing which two traingles out of the 8 visible types would be easy to deduce the area of kite ? | ||
| + | *Question Corner: | ||
| + | # Recall the two formulae that you know to find the area of a triangle. | ||
===Activity No # === | ===Activity No # === | ||
Revision as of 17:07, 19 December 2013
| Philosophy of Mathematics |
While creating a resource page, please click here for a resource creation checklist.
Concept Map
Error: Mind Map file kite.mm not found
Textbook
To add textbook links, please follow these instructions to: (Click to create the subpage)
Additional Information
Useful websites
- http://www.transum.org/software/Fun_Maths/kite. This website has good description and activities about kites.
Reference Books
Teaching Outlines
Concept # 1. A Kite and its properties
Learning objectives
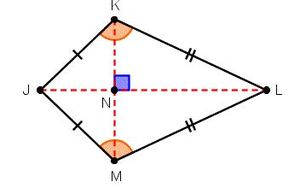
- A kite is a quadrilateral with two distinct pairs of adjacent sides that are congruent.
- The two pairs of congruent sides meet at two different points.
- A kite can also be described as a quadrilateral with an axis of symmetry along one of its diagonals.
- Kites have a couple of properties that will help us identify them from other quadrilaterals.
- The diagonals of a kite meet at a right angle.
- Kites have exactly one pair of opposite angles that are congruent.
- Diagnols intersect at right angles.
<K = <M. This is the only pair of congruent angles because <J and <L have different measures.
Notes for teachers
- A kite is sometimes also known as a deltoid.
- A kite, may be either convex or concave, but the word "kite" is often restricted to the convex variety. A concave kite is sometimes called a "dart" or "arrowhead".
Activity No # 1. Paper kite
- Estimated Time: 30 minutes.
- Materials/ Resources needed :
- A4 sheet of paper.
- Prerequisites/Instructions, if any
- Neat paper folding skills.
- Ability to follow instructions.
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
This activity has been taken from the website : http://www.transum.org/software/Fun_Maths/kite/
- Process:
- Fold an A4 sheet of paper as shown in the figures to make a kite.
- Developmental Questions:
- Which is the figure formed ?
- What is special about this quadrilateral ?
- How many sides does a kite have ?
- Are all sides equal ?
- Mark the diagnols ? What do you notice about them ?
- Evaluation:
- Were the students able dto recognise the properties of a kite.
- Question Corner
- Compare kite with other quadrilaterals and make a list of similarities and differences between them.
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept #2. Measurements in a kite
Learning objectives
- A kite has two pairs of congruent sides.
- Its diagnols intersect at right angles.
- The sum of its four sides would be its perimetre.
- Its area is given by the formula
Notes for teachers
Activity No # Deriving formula for area of a kite
- Estimated Time : 30 minutes.
- Materials/ Resources needed: Laptop, geogebra file, projector and a pointer.
- Prerequisites/Instructions, if any
- They should know a kite and its properties.
- The students should know the concept of an area.
- They should know the formula to find the area of a triangle.
- Multimedia resources: Laptop
- Website interactives/ links/ / Geogebra Applets
- Process:
- The teacher can project the geogebra file on kite.
- Show them that a kite is made of two isosceles traingles.
- Sum of areas of these two triangles would be the area of the kite.
- Developmental Questions
- What is a kite /
- What are the properties of a kite.
- What other figures can you see in a kite ?
- What types of triangles are these ?
- Identify the two isosceles triangles ?
- What is the formula to find the area of a triangle ?
- Evaluation:
- Choosing which two traingles out of the 8 visible types would be easy to deduce the area of kite ?
- Question Corner:
- Recall the two formulae that you know to find the area of a triangle.
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept # 3. Construction of a kite
Learning objectives
Notes for teachers
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept #
Learning objectives
Notes for teachers
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Hints for difficult problems
Project Ideas
Math Fun
Usage
Create a new page and type {{subst:Math-Content}} to use this template