Difference between revisions of "Cyclic quadrilateral"
Jump to navigation
Jump to search
| Line 169: | Line 169: | ||
Laptop, geogebra file, projector and a pointer. | Laptop, geogebra file, projector and a pointer. | ||
*Prerequisites/Instructions, if any | *Prerequisites/Instructions, if any | ||
| − | # | + | # A cyclic quadrilateral and its properties. |
| − | # | + | # The linear pair and exterior angle theorem. |
| − | # | + | # The circle theorem (Angle at centre = double the angle at the circumference) |
*Multimedia resources: Laptop | *Multimedia resources: Laptop | ||
*Website interactives/ links/ / Geogebra Applets: | *Website interactives/ links/ / Geogebra Applets: | ||
Revision as of 13:39, 6 January 2014
| Philosophy of Mathematics |
While creating a resource page, please click here for a resource creation checklist.
Concept Map
Error: Mind Map file Cyclic_quadrilateral.mm not found
Textbook
To add textbook links, please follow these instructions to: (Click to create the subpage)
Additional Information
Useful websites
Reference Books
Teaching Outlines
Concept # 1. Cyclic quadrilateral and its properties
Learning objectives
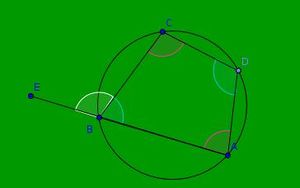
- A quadrilateral ABCD is called cyclic if all of its four vertices lie on a circle.
- In a cyclic quadrilateral the sum of opposite interior angles is 180 degrees.
- If the sum of a pair of opposite angles of a quadrilateral is 180, the quadrilateral is cyclic.
- In a cyclic quadrilateral the exterior angle is equal to interior opposite angle.
Notes for teachers
Activity#1 Cyclic quadrilateral
- Estimated Time 10 minutes
- Materials/ Resources needed: Laptop, geogebra file, projector and a pointer.
- Prerequisites/Instructions, if any
- Circle and quadrilaterals should have been introduced.
- Multimedia resources : Laptop
- Website interactives/ links/ / Geogebra Applets
- Process:
- The teacher can recall the concept of a circle, quadrilateral, circumcircle.
- Can explain a cyclic quadrilateral and show the geogebra applet.
- Move points, the vertices of the quadrilateral and let the students observe the sum of opposite interior angles.
Developmental Questions:
- What two figures do you see in the figure ?
- Name the vertices of the quadrilateral.
- Where are all the 4 vertices situated ?
- Name the opposite interior angles of the quadrilateral.
- What do you observe about them.
- Evaluation:
- Compare the cyclic quadrilateral to circumcircle.
- Question Corner
- Can all quadrilaterals be cyclic ?
- What are the necessary conditions for a quadrilateral to be cyclic ?
Activity No # 2.Properties of a Cyclic quadrilateral
- Estimated Time: 45 minutes
- Materials/ Resources needed
coloured paper, pair of scissors, sketch pen, carbon paper, geometry box
- Prerequisites/Instructions, if any
- In a cyclic quadrilateral the sum of opposite interior angles is 180 degrees.
- In a cyclic quadrilateral the exterior angle is equal to interior opposite angle
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
This activity has been taken from the website http://mykhmsmathclass.blogspot.in/2007/11/class-ix-activity-16.html
- Process:
- Draw a circle of any radius on a coloured paper and cut it.
- Paste the circle cut out on a rectangular sheet of paper.
- By paper folding get chords AB, BC, CD and DA in order.
- Draw AB, BC, CD & DA. A cyclic quadrilateral ABCD is obtained.
- Produce AB to form a ray AE such that exterior angle CBE is formed.
- Make a replica of cyclic quadrilateral ABCD using carbon paper.
- Cut the replica into 4 parts such that each part contains one angle .
- Draw a straight line on a paper.
- Place the two opposite angles, angle BAD and angle BCD adjacent to each other on the straight line.Write the observation.
- Place other two opposite angles, angle ABC and angle ADC adjacent to each other on the straight line . Write the observation.
- Make a replica of angle ADC and place it on angle CBE . Write the observation.
Developmental Questions:
- How do you take radius ?
- What is the circumference ?
- What is a chord ?
- What is a quadrilateral ?
- Where are all four vertices of a quadrilateral located ?
- What part are we trying to cut and compare ?
- What can you infer ?
- Evaluation:
- Angle BAD and angle BCD, when placed adjacent to each other on a straight line, completely cover the straight angle.What does this mean ?
- Angle ABC and angle ADC, when placed adjacent to each other on a straight line, completely cover the straight angle.What can you conclude ?
- Compare angle ADC with angle CBE.
- Question Corner:
Name the two properties of cyclic quarilaterals.
Concept # 2.Construction of cyclic quadrilateral
Learning objectives
- Ability to construct a cyclic quadrilateral accurately .
Notes for teachers
Activity No # Constructing a cyclic quadrilateral
- Estimated Time: 40 minutes.
- Materials/ Resources needed:
- Laptop, geogebra file, projector and a pointer.
- Students constructing materials, the geometry box.
- white papers.
- Prerequisites/Instructions, if any
- Sufficient knowledge regarding construction of perpendicular lines, bisectors, angles and circle.
- Multimedia resources : Laptop
- Website interactives/ links/ / Geogebra Applets: For step by step illustration of cyclic quadrilateral construction please refer to the website: http://www.matrusrieppower.net/Constructionoftriangleandcyclicquadrilateral.html.
- Process:
- The teacher can do this activity after introducing the concept and properties of cyclic quadrilateral.
- She can project the file and let students watch it carefully.
- After watching discuss the steps of construction and the purpose of each step so that the students can appreciate the sequence of construction steps.
- Then ask the students to actually construct a cyclic quadrilateral for the given measures.
- Developmental Questions:
- What is a cyclic quadrilateral ? Why is it called so ?
- Name the measuring parameters of it ?
- What measures are given for its construction ?
- Explain the steps involved in determing the radius of the required circle ?
- What do the measures of the arcs specify ?
- Evaluation:
- Were the students able to justify the sequence of steps involved ?
- Question Corner:
- Can you draw a circle first and then the quadrilateral ? Why not so ?
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept # 3. Theorems on cyclic quadrilaterals
Learning objectives
- Both pairs of opposite angles of a cyclic quadrilateral are supplementary.
- When one side of a cyclic quadrilateral is produced, the exterior angle so formed is equal to the interior opposite angle.
Converse theorems:
- Suppose a quadrilateral is such that the sum of two opposite angles is a straight angle, them the quadrilateral is cyclic.
- If the exterior angle of a quadrilateral is equal to the interior opposite angle, then the quadrilateral is cyclic.
Notes for teachers
Activity No 1. Theorems
- Estimated Time : 40 minutes.
- Materials/ Resources needed:
Laptop, geogebra file, projector and a pointer.
- Prerequisites/Instructions, if any
- A cyclic quadrilateral and its properties.
- The linear pair and exterior angle theorem.
- The circle theorem (Angle at centre = double the angle at the circumference)
- Multimedia resources: Laptop
- Website interactives/ links/ / Geogebra Applets:
- Process:
- The teacher can project the geogebra file and prove the theorems.
- Developmental Questions:
- How many angles does a cyclic quadrilateral have ?
- Name the opposite angles of it.
- Name the minor arc.
- Recall the angle -arc theorem.
- What is the total angle at the centre of a circle ?
- Name the angles at the centre of the circle.
- What is the sum of those two angles ?
- How can you show that <b and <d are supplementary from above observations ?
- Evaluation;
- What is the converse of this theorem.
- Question Corner;
- Write down the steps to prove the converse of this theorem.
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Hints for difficult problems
Project Ideas
Math Fun
Usage
Create a new page and type {{subst:Math-Content}} to use this template