Difference between revisions of "Solution"
m (added Category:Quadratic Equations using HotCat) |
|||
| (6 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
=Hints for difficult problems = | =Hints for difficult problems = | ||
#If P & q are the roots of the equation <math>2a^2-4a+1=0</math> find the value of | #If P & q are the roots of the equation <math>2a^2-4a+1=0</math> find the value of | ||
| − | <math>p^3+q^3</math> | + | <math>p^3+q^3</math><br> |
'''Pre requisites''': | '''Pre requisites''': | ||
#Standard form of quadratic equation | #Standard form of quadratic equation | ||
| Line 27: | Line 27: | ||
'''to back to concept page''' | '''to back to concept page''' | ||
[[http://karnatakaeducation.org.in/KOER/en/index.php/Quadratic_Equations#Hints_for_difficult_problems| quadratic equation problems]]<br> | [[http://karnatakaeducation.org.in/KOER/en/index.php/Quadratic_Equations#Hints_for_difficult_problems| quadratic equation problems]]<br> | ||
| − | 2.The altitude of a triangle is 6cm greter than its base. If its area is 108cmsq .Find its base.<br> | + | 2.'''The altitude of a triangle is 6cm greter than its base. If its area is 108cmsq .Find its base'''.<br> |
[[solution]]<br> | [[solution]]<br> | ||
Statement: Solving problem based on quadratic equations.<br> | Statement: Solving problem based on quadratic equations.<br> | ||
| Line 51: | Line 51: | ||
*Using Formula<br> | *Using Formula<br> | ||
*Using Graph<br> | *Using Graph<br> | ||
| − | '''back to concept page''' | + | |
| − | + | '''3.Solve x2 – 4x – 8 = 0. By completing the square.'''<br> | |
| + | '''Interpretation of the problem:'''<br> | ||
| + | |||
| + | #Is it a quadratic equation? | ||
| + | #Knowledge about coefficients of the variable. | ||
| + | #Knowledge of steps for completing the given equation as square. | ||
| + | #Knowledge of root. | ||
| + | |||
| + | '''Different approaches to solve:''' | ||
| + | #Factorization Method (sometimes in few ex as x2+6x-7=0) | ||
| + | #Completing the square. | ||
| + | |||
| + | As noted above, this quadratic does not factor, so I can't solve the equation by factoring. And they haven't given me the quadratic in a form that is ready to square-root. But there is a way for me to manipulate the quadratic to put it into that form, and then solve. It works like this:<br> | ||
| + | |||
| + | |||
| + | 1) First, I put the loose number “8” on the other side of the equation: <br> | ||
| + | x2 – 4x – 8 = 0 <br> | ||
| + | x2 – 4x = 8 <br> | ||
| + | |||
| + | 2) Then I look at the coefficient of the x-term, which is –4 in this case. I take half of this number (including the sign), giving me –2. Then I square this value to get +4, and add this squared value to both sides of the equation:<br> | ||
| + | |||
| + | x2 – 4x + 4 = 8 + 4 <br> | ||
| + | x2 – 4x + 4 = 12 <br> | ||
| + | |||
| + | 3) This process creates a quadratic that is a perfect square, and factoring gives me: <br> | ||
| + | (x – 2)2 = 12 <br> | ||
| + | Tip : I know it's a "minus two" inside the parentheses because half of –4 is –2. If you note the sign when you're finding one-half of the coefficient, then you won't mess up the sign when you're converting to squared-binomial form.<br> | ||
| + | |||
| + | |||
| + | 4) Now I can square-root both sides of the equation, simplify, and solve:<br> | ||
| + | (x – 2)2 = 12<br> | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | '''For each approach:''' | ||
| + | '''Prior knowledge:''' | ||
| + | |||
| + | *About quadratic equation | ||
| + | *About co-efficient’s | ||
| + | *Comparing the equation with standard form | ||
| + | *Dividing and squaring the value of ‘b’ | ||
| + | |||
| + | '''Gap identification:''' | ||
| + | Recalling the standard equation.<br> | ||
| + | Identifying the values of a,b,c in given equation <br> | ||
| + | Dividing and squaring <br> | ||
| + | |||
| + | '''Algorithm''' | ||
| + | |||
| + | 1. Equating the equation to zero or standard form. | ||
| + | 2. Translating loose number “ c” i.e. constant on the other side of the equation | ||
| + | 3. Making half and squaring the co-efficient of variable (x). | ||
| + | 4. Add the square on both sides of equation. | ||
| + | 5. Put in the complete square form. (a+b)2 or (a-b) 2 | ||
| + | 6. Find the value of variable ‘x’ /square-roots of the variable | ||
| + | |||
| + | '''Activity 1''' | ||
| + | Solving Quadratic Equations by Completing the Square<br> | ||
| + | |||
| + | https://www.youtube.com/watch?v=bclm1tJB-3g <br> | ||
| + | |||
| + | Note for teachers: Unless you're told that you have to use completing the square, you will probably not use this method, in actual practice, when solving quadratic equations. Either some other method (such as factoring) will be obvious and quicker, or else the Quadratic Formula will be easier to use. However, if your class covered completing the square, you should expect to be required to show that you can complete the square to solve a quadratic on the next test. | ||
| + | For more examples refer | ||
| + | |||
| + | http://www.purplemath.com/modules/sqrquad.htm | ||
| + | |||
| + | '''Activity 2''' | ||
| + | '''Worksheet for Students''' | ||
| + | |||
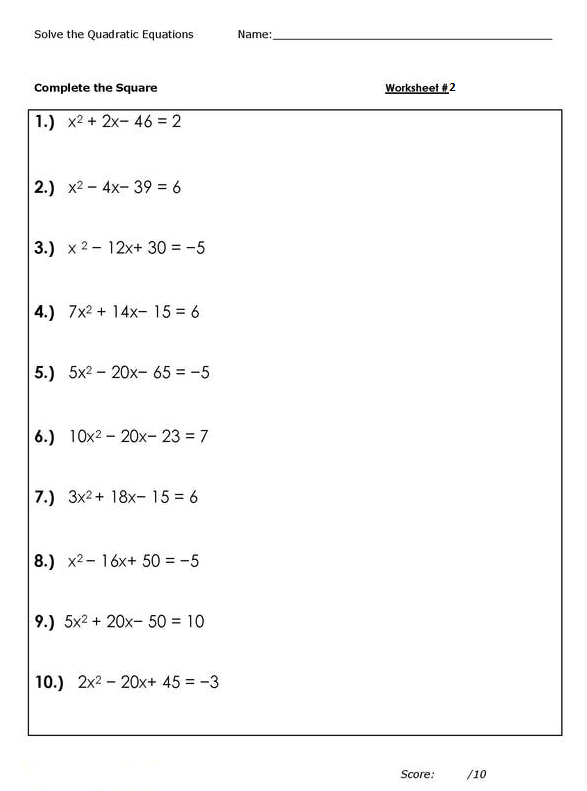
| + | [[File:Worksheet1.jpeg]] | ||
| + | |||
| + | [[File:Worksheet2.jpeg]] | ||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | |||
| + | '''back to concept page'''[http://karnatakaeducation.org.in/KOER/en/index.php/Quadratic_Equations#Hints_for_difficult_problems "solution"]<br> | ||
| + | |||
| + | [[Category:Quadratic Equations]] | ||
Latest revision as of 09:49, 30 October 2019
Hints for difficult problems
- If P & q are the roots of the equation find the value of
Pre requisites:
- Standard form of quadratic equation
- Formula to find the sum & product of quadratic equation
- Knowledge of using appropriate identity
Interpretation of the Problem:
- Compare the equation with standard form and identify the values of a,b,c
- To find the sum formformof the roots of the quadratic equation using the formula
- To find the product of the roots of the equation
- Using the identity & rewriting as
- Substitute the values of m+n & mn in
- Simplification
Concepts:
- Formula to find the sum and product of the roots of the quadratic equation
- Identity
Algorithm:
Consider the equation
Here a=2,b=-4 & c=1
If p & q are the roots of the quadratic equation then
Therefore,
=
=8-3
=5
to back to concept page
[quadratic equation problems]
2.The altitude of a triangle is 6cm greter than its base. If its area is 108cmsq .Find its base.
solution
Statement: Solving problem based on quadratic equations.
solution
- Interpretation of the problem:
* Converting data in to eqn.
*Knowledge about area of a triangle.
*knowledge of the formula of area of triangle.
*Methods of finding the roots of the eqn.
*Methods of finding the roots of the - Different approches to solve the problem:
*Factorisation - Using formula
- using graph
- Concept used:Forming the eqn. 216=x(x+6)
216=x2+6x
x2 +6x -216=0
Substitution: x 2 +18x-12x -216=0
Simplification: x(x+18)-12(x+18)=0
(x+18)( x-12)=0
(x+18)=0 (x-12)=0
x=-18, x=12
.
- Base=12cm,
Altitude=x+6
=12+6=18cm.
Prior Knowledge -
- Methods of solving the Eqn
- Factorisation
- Using Formula
- Using Graph
3.Solve x2 – 4x – 8 = 0. By completing the square.
Interpretation of the problem:
- Is it a quadratic equation?
- Knowledge about coefficients of the variable.
- Knowledge of steps for completing the given equation as square.
- Knowledge of root.
Different approaches to solve:
- Factorization Method (sometimes in few ex as x2+6x-7=0)
- Completing the square.
As noted above, this quadratic does not factor, so I can't solve the equation by factoring. And they haven't given me the quadratic in a form that is ready to square-root. But there is a way for me to manipulate the quadratic to put it into that form, and then solve. It works like this:
1) First, I put the loose number “8” on the other side of the equation:
x2 – 4x – 8 = 0
x2 – 4x = 8
2) Then I look at the coefficient of the x-term, which is –4 in this case. I take half of this number (including the sign), giving me –2. Then I square this value to get +4, and add this squared value to both sides of the equation:
x2 – 4x + 4 = 8 + 4
x2 – 4x + 4 = 12
3) This process creates a quadratic that is a perfect square, and factoring gives me:
(x – 2)2 = 12
Tip : I know it's a "minus two" inside the parentheses because half of –4 is –2. If you note the sign when you're finding one-half of the coefficient, then you won't mess up the sign when you're converting to squared-binomial form.
4) Now I can square-root both sides of the equation, simplify, and solve:
(x – 2)2 = 12
For each approach: Prior knowledge:
- About quadratic equation
- About co-efficient’s
- Comparing the equation with standard form
- Dividing and squaring the value of ‘b’
Gap identification:
Recalling the standard equation.
Identifying the values of a,b,c in given equation
Dividing and squaring
Algorithm
1. Equating the equation to zero or standard form. 2. Translating loose number “ c” i.e. constant on the other side of the equation 3. Making half and squaring the co-efficient of variable (x). 4. Add the square on both sides of equation. 5. Put in the complete square form. (a+b)2 or (a-b) 2 6. Find the value of variable ‘x’ /square-roots of the variable
Activity 1
Solving Quadratic Equations by Completing the Square
https://www.youtube.com/watch?v=bclm1tJB-3g
Note for teachers: Unless you're told that you have to use completing the square, you will probably not use this method, in actual practice, when solving quadratic equations. Either some other method (such as factoring) will be obvious and quicker, or else the Quadratic Formula will be easier to use. However, if your class covered completing the square, you should expect to be required to show that you can complete the square to solve a quadratic on the next test. For more examples refer
http://www.purplemath.com/modules/sqrquad.htm
Activity 2 Worksheet for Students
back to concept page"solution"







}](https://en.wikipedia.org/api/rest_v1/media/math/render/svg/e2eea7333ac882aad6301def702540ebe6461b95)