Formation of a triangle

Objectives

• Understand formation of triangles
• Recognize elements of triangle
• Introduce concepts of exterior angle.

Introduction to a triangle.ggb

Estimated Time

30 minutes

Prerequisites/Instructions, prior preparations, if any

Prior knowledge of point, lines, angles, parallel lines

Materials/ Resources needed

• Digital : Computer, geogebra application, projector.
• Non digital : Worksheet and pencil
• Geogebra files : “Introduction to a triangle.ggb”

Process (How to do the activity)

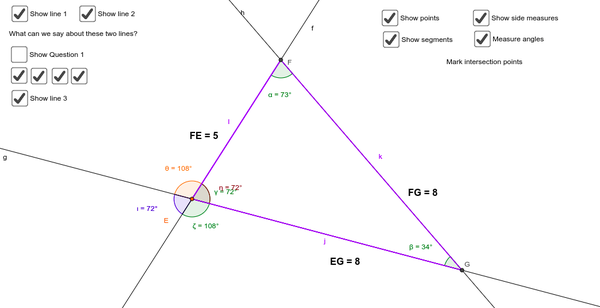
• Use the geogebra file to illustrate.
• How many lines are there? Are the lines meeting?
• Are the two lines parallel? How can you say they are parallel or not?
• How many angles are formed at the point of intersection?
• What is the measure of the total angle at the point of intersection of two lines?
• Of the four angles formed which of the angles are equal? What are they called?
• Do the three intersecting lines enclose a space? How does it look? It is called a triangle.
• What are the points of intersection of these three lines called?
• The line segments forming the triangle are called sides.
• How many angles are formed when three lines intersect with each other?
• How many angles are enclosed by the triangle?

Evaluation at the end of the activity

• Can there be a closed figure with less than three sides?
• Can the vertices of the triangle be anywhere on a plane?
• What will happen if the three vertices are collinear?