Difference between revisions of "Triangles"

From Karnataka Open Educational Resources
Jump to navigation Jump to search
Line 33: Line 33:
 
# http://www.regentsprep.org/regents/math/geometry/GPB/theorems.htm
 
# http://www.regentsprep.org/regents/math/geometry/GPB/theorems.htm
 
A good website for quick reference of all theorems in geometry. Suitable for both students and teachers.
 
A good website for quick reference of all theorems in geometry. Suitable for both students and teachers.
 +
# Click [http://karnatakaeducation.org.in/KOER/en/index.php/Classification_of_triangles here] for notes on types of triangles.
 +
# Click [http://karnatakaeducation.org.in/KOER/en/index.php/Classification_of_triangles here] for notes on types of triangles.
 +
  
 
==Reference Books==
 
==Reference Books==
Line 40: Line 43:
 
==Concept #1 A triangle and its properties==
 
==Concept #1 A triangle and its properties==
 
===Learning objectives===
 
===Learning objectives===
 +
# A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges
 +
which are line segments. 
 +
# It is the polygon with the least number of sides.
 
# A triangle can be defined as a polygon which has 3 sides, 3 angles and 3 vertices.
 
# A triangle can be defined as a polygon which has 3 sides, 3 angles and 3 vertices.
 
# The sum of any two sides is always greater than the third side.
 
# The sum of any two sides is always greater than the third side.
Line 45: Line 51:
 
# The sum of all 3 angles in any triangle is always 180 degrees which is called the angle sum property of a triangle.
 
# The sum of all 3 angles in any triangle is always 180 degrees which is called the angle sum property of a triangle.
 
===Notes for teachers===
 
===Notes for teachers===
−
''These are short notes that the teacher wants to share about the concept, any locally relevant information, specific instructions on what kind of methodology used and common misconceptions/mistakes.''
+
[''These are short notes that the teacher wants to share about the concept, any locally relevant information, specific instructions on what kind of methodology used and common misconceptions/mistakes.'']
−
 
+
# A triangle PQR consists of all the points on the line segment PQ,QR and RP. The three line segments, PQ, QR and RP that form
−
Triangle is the most basic polygon shape.  Triangles are classified on the basis of their angles and sides.
+
the triangle PQ, are called the sides of the triangle PQR.
 +
# A triangle has three angles. In figure, the three angles are ∠PQR ∠QRP and ∠RPQ
 +
# A triangle has six parts, namely, three sides,PQ QRand RP.Three angles ∠PQR ∠QRP and ∠RPQ. These are also known as the elements of a triangle.
 +
# The point of intersection of the sides of a triangle is known as its vertex. In figure, the three vertices are P, Q and R. In a triangle, an angle is formed at the vertex. Since it has three vertices, so three angles are formed. The word triangle =tri + angle ‘tri’ means three. So, triangle means closed figure of straight lines having three angles.
  
−
Click [http://karnatakaeducation.org.in/KOER/en/index.php/Classification_of_triangles here] for notes on types of triangles.
+
===Activity No # 1 Make your triangle ===
−
 
 
−
===Activity No #1 - Make your triangle ===
 
 
{| style="height:10px; float:right; align:center;"
 
{| style="height:10px; float:right; align:center;"
 
|<div style="width:150px;border:none; border-radius:10px;box-shadow: 5px 5px 5px #888888; background:#f5f5f5; vertical-align:top; text-align:center; padding:5px;">
 
|<div style="width:150px;border:none; border-radius:10px;box-shadow: 5px 5px 5px #888888; background:#f5f5f5; vertical-align:top; text-align:center; padding:5px;">
 
''[http://www.karnatakaeducation.org.in/?q=node/305 Click to Comment]''</div>
 
''[http://www.karnatakaeducation.org.in/?q=node/305 Click to Comment]''</div>
 
|}
 
|}
−
* '''Estimated Time''' - 40 minutes
+
* Estimated Time - 40 minutes
−
* '''Materials/ Resources needed'''
+
* Materials/ Resources needed; Paper, pencil, and scale.
−
* '''Prerequisites/Instructions, if any'''
+
* Prerequisites/Instructions, if any:
−
* '''Multimedia resources'''
+
# The students should know points and line segments.
−
* '''Website interactives/ links/ Geogebra Applets'''
+
* Multimedia resources
−
* '''Process (How to do the activity)'''
+
* Website interactives/ links/ Geogebra Applets
−
Mark three non-collinear point P, Q and R on a paper. Join these points in all possible ways. The segments are PQ, QR and RP. A simple close curve formed by these three segments is called a triangle. It is named in one of the following ways.
+
* Process (How to do the activity):
−
+
# Mark three non-collinear point P, Q and R on a paper.  
−
Triangle PQR or Triangle PRQ or Triangle QRP or Triangle RPQ or Triangle RQP .
+
# Join these points in all possible ways. The segments are PQ, QR and RP.  
 +
# A simple close curve formed by these three segments is called a triangle. It is named in one of the following ways.
 +
# Triangle PQR or Triangle PRQ or Triangle QRP or Triangle RPQ or Triangle RQP .
  
 
[[Image:KOER%20Triangles_html_m55b3a2cf.png|picture of equilateral triangle]] PQR
 
[[Image:KOER%20Triangles_html_m55b3a2cf.png|picture of equilateral triangle]] PQR
 
   
 
   
−
* '''Developmental Questions (What discussion questions)'''
+
* Developmental Questions (What discussion questions);
−
A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges
 
−
which are line segments.  In fact, it is the polygon with the least number of sides.
 
−
 
 
−
A triangle PQR consists of all the points on the line segment PQ,QR and RP. The three line segments, PQ, QR and RP that form
 
−
the triangle PQ, are called the sides of the triangle PQR.
 
  
−
How many angles? A triangle has three angles. In figure, the three angles are ∠PQR ∠QRP and ∠RPQ
 
−
A triangle has six parts, namely, three sides,PQ QRand RP.Three angles ∠PQR ∠QRP and ∠RPQ. These are also known
 
−
as the elements of a triangle.
 
  
−
What are the intersection points of line segments?
 
−
The point of intersection of the sides of a triangle is known as its vertex. In figure, the three vertices are P, Q and R. In a triangle, an angle is formed at the vertex. Since it has three vertices, so three angles are formed. The word triangle =tri + angle ‘tri’ means three. So, triangle means closed figure of straight lines having three angles.
 
  
  

Revision as of 10:54, 26 December 2013

The Story of Mathematics

Philosophy of Mathematics

Teaching of Mathematics

Curriculum and Syllabus

Topics in School Mathematics

Textbooks

Question Bank

While creating a resource page, please click here for a resource creation checklist.

Concept Map

Error: Mind Map file Triangles .mm not found


Textbook

To add textbook links, please follow these instructions to: (Click to create the subpage)

Additional Information

Useful websites

  1. All about triangles

This is a reference website for types and classification off triangles

  1. http://www.regentsprep.org/regents/math/geometry/GPB/theorems.htm

A good website for quick reference of all theorems in geometry. Suitable for both students and teachers.

  1. Click here for notes on types of triangles.
  2. Click here for notes on types of triangles.


Reference Books

Teaching Outlines

Concept #1 A triangle and its properties

Learning objectives

  1. A triangle is one of the basic shapes of geometry: a polygon with three corners or vertices and three sides or edges

which are line segments.

  1. It is the polygon with the least number of sides.
  2. A triangle can be defined as a polygon which has 3 sides, 3 angles and 3 vertices.
  3. The sum of any two sides is always greater than the third side.
  4. The angle opposite to longest side is the largest.
  5. The sum of all 3 angles in any triangle is always 180 degrees which is called the angle sum property of a triangle.

Notes for teachers

[These are short notes that the teacher wants to share about the concept, any locally relevant information, specific instructions on what kind of methodology used and common misconceptions/mistakes.]

  1. A triangle PQR consists of all the points on the line segment PQ,QR and RP. The three line segments, PQ, QR and RP that form

the triangle PQ, are called the sides of the triangle PQR.

  1. A triangle has three angles. In figure, the three angles are ∠PQR ∠QRP and ∠RPQ
  2. A triangle has six parts, namely, three sides,PQ QRand RP.Three angles ∠PQR ∠QRP and ∠RPQ. These are also known as the elements of a triangle.
  3. The point of intersection of the sides of a triangle is known as its vertex. In figure, the three vertices are P, Q and R. In a triangle, an angle is formed at the vertex. Since it has three vertices, so three angles are formed. The word triangle =tri + angle ‘tri’ means three. So, triangle means closed figure of straight lines having three angles.

Activity No # 1 Make your triangle

  • Estimated Time - 40 minutes
  • Materials/ Resources needed; Paper, pencil, and scale.
  • Prerequisites/Instructions, if any:
  1. The students should know points and line segments.
  • Multimedia resources
  • Website interactives/ links/ Geogebra Applets
  • Process (How to do the activity):
  1. Mark three non-collinear point P, Q and R on a paper.
  2. Join these points in all possible ways. The segments are PQ, QR and RP.
  3. A simple close curve formed by these three segments is called a triangle. It is named in one of the following ways.
  4. Triangle PQR or Triangle PRQ or Triangle QRP or Triangle RPQ or Triangle RQP .

picture of equilateral triangle PQR

  • Developmental Questions (What discussion questions);



  • Evaluation (Questions for assessment of the child)
  • Question Corner

Activity No # 2 - Types of triangles - Identifying and naming

Part 1

  • Estimated time - 20 minutes
  • Materials/ Resources needed
  • Prerequisites/Instructions, if any
  • Multimedia resources

KOER Triangles html m27d3a9c5.png

  • Website interactives/ links/ Geogebra Applets
  • Process (How to do the activity)

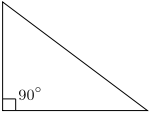
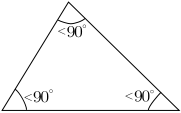
Identify and name the triangles in the above figure.

  • Evaluation
  1. Is it possible to construct a triangle with 3 collinear points?
  2. Is it possible to construct a triangle whose sides are 3cm, 4cm and 9cm. Why?.

Part 2

  • Estimated time - 20 minutes
  • Materials/ Resources needed
  • Prerequisites/Instructions, if any
  • Multimedia resources

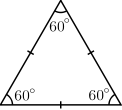
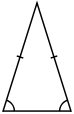
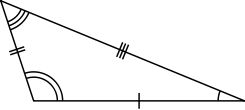
Look at the following images below

Equilateral Triangle Isosceles triangle Scalene triangle
Right triangle Obtuse triangle Acute triangle
  • Website interactives/ links/ Geogebra Applets
  • Process (How to do the activity)

Identify and name the triangles in the above figure.

  • Evaluation
  1. Can a scalene triangle also be a right-angled triangle ? If yes can you draw one ?

Concept #2 - Properties and Theorems: Thales Theorem

Learning objectives

Notes for teachers

  1. The GeoGebra file below verifies the Thales theorem

These are short notes that the teacher wants to share about the concept, any locally relevant information, specific instructions on what kind of methodology used and common misconceptions/mistakes.

Activity No #

  • Estimated Time
  • Materials/ Resources needed
  • Prerequisites/Instructions, if any
  • Multimedia resources
  • Website interactives/ links/ Geogebra Applets
  • Process (How to do the activity)
  • Developmental Questions (What discussion questions)
  • Evaluation (Questions for assessment of the child)
  • Question Corner


Activity No #

  • Estimated Time
  • Materials/ Resources needed
  • Prerequisites/Instructions, if any
  • Multimedia resources
  • Website interactives/ links/ Geogebra Applets
  • Process (How to do the activity)
  • Developmental Questions (What discussion questions)
  • Evaluation (Questions for assessment of the child)
  • Question Corner


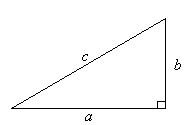
Concept #3 - Properties and Theorems: Pythagoras Theorem

Learning objectives

Notes for teachers

Pythagoras' Theorem was discovered by Pythagoras, a Greek mathematician and philosopher who lived between approximately 569 BC and 500 BC. Pythagoras' Theorem states that:

In any right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. That is:

KOER Triangles html m2f096af4.png KOER Triangles html 4bd439df.png

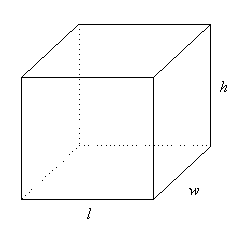
Pythagoras' Theorem in Three Dimensions A three-dimensional object can be described by three measurements - length, width and height. 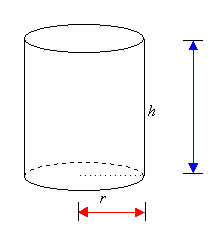KOER Triangles html 679fe2f6.pngKOER Triangles html m570261d2.png We can use Pythagoras' Theorem to find the length of the longest straw that will fit inside the box or cylinder.

Activity No #

  • Estimated Time
  • Materials/ Resources needed
  • Prerequisites/Instructions, if any
  • Multimedia resources
  • Website interactives/ links/ Geogebra Applets
  • Process (How to do the activity)
  • Developmental Questions (What discussion questions)
  • Evaluation (Questions for assessment of the child)
  • Question Corner


Activity No #

  • Estimated Time
  • Materials/ Resources needed
  • Prerequisites/Instructions, if any
  • Multimedia resources
  • Website interactives/ links/ Geogebra Applets
  • Process (How to do the activity)
  • Developmental Questions (What discussion questions)
  • Evaluation (Questions for assessment of the child)
  • Question Corner

Hints for difficult problems

Project Ideas

Math Fun

Usage

Create a new page and type {{subst:Math-Content}} to use this template

Retrieved from "https://karnatakaeducation.org.in/KOER/en/index.php?title=Triangles&oldid=8303"