Angles
Concept Plan
| Philosophy of Mathematics |
While creating a resource page, please click here for a resource creation checklist.
Textbook
To add textbook links, please follow these instructions to: (Click to create the subpage)
Additional Information
Useful websites
Reference Books
Teaching Outlines
Concept #1.What is an angle ?
Learning objectives
Notes for teachers
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept #2. Using a Protractor- Measuring an angle
Learning objectives
Notes for teachers
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept #3.Types of angles
Learning objectives
Notes for teachers
Activity No #1.Crazy Angles using Geogebra
- Estimated Time: 40 minutes
- Materials/ Resources needed: Laptop, geogebra file, projector and a pointer.
- Prerequisites/Instructions, if any:
- The students should have a basic understanding about point, rays, line segments and vertex.
- They should know how angles are formed.
- They should know that angles are measured in units called degrees. 360 ° is a full rotation (a circle)
- They should know to use a protractor and measure the angles.
- They should know the meaning of terms acute, obtuse, straight, reflex, and complete angles.
- Multimedia resources; Laptop
- Website interactives/ links/ / Geogebra Applets
- Process:
- The teacher should recaptulate the concept of a point, line segment, ray, vertex and angles.
- The teacher should show how angles are formed.
- Discuss the concept of cartesian plane, X and Y axes, rotation, and how it relates to angles.
- Demonstrate how to measure angles using a protractor.
- Define and illustrate the classification of the types of angles—acute, obtuse, right, straight zero and complete angles.
- In the succeeding class give the students protractors and let them have enough practise measuring and classifying angles.
Developmental Questions:
- What is a point ?
- A minimum of how many points are needed to define a line segment ?
- A minimum of how many points are needed to form an angle ?
- Name the line segments from the figure.
- What is a vertex ?
- How many rays /line segments are needed to form an angle ?
- Name the vertex at which the angle is formed
- Name the angle .
- Name the type of angle formed.
- Evaluation:
- Assess the students knowledge of angles by projecting different types of angles and asking them to name
- What are the characteristics of an acute angle ?
- What are the characteristics of an obtuse angle?
- What are the characteristics of a right angle
- Evaluate if the students have understood that :
- An angle is formed where 2 lines meet at a point.
- A right angle looks like a corner of a square or a rectangle.
- An acute angle is narrower than a right angle.
- An obtuse angle is wider than a right angle.
- Question Corner:
- What is an angle ?
- Where do you name an angle ?
- How do you identify different types of angles in 2-dimensional figures?
- How do angles help to classify 2-dimensional figures?
- Are angles <ABA' and <A'BA the same ? Justify
- Differentiate between the zero angle and a complete angle.
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept # 4. Angle constructions
Learning objectives
Notes for teachers
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept # 5. Angle bisector-Its construction
Learning objectives
Notes for teachers
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept # 6. Pairs of angles
Learning objectives
Notes for teachers
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Concept # 7.Angles formed when lines are cut by a transversal
Learning objectives
Notes for teachers
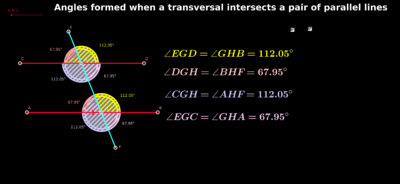
Activity No # 1.Angles formed when a transversal intersects parallel lines
*Estimated Time : 40 minutes
*Materials/ Resources needed :Laptop, geogebra file, projector and pointer.
*Prerequisites/Instructions, if any :
- The students should have prior knowledge of parallel lines , transversal, angles and types of angles formed when a pair of parallel lines are intersected by a transversal.
- They should know what the terms interior, exterior, adjacent, alternate, consecutive, congruent, linear and corresponding mean.
- Students should know the definition of complementary angles, supplementary angles, and congruent angles.
*Multimedia resources:
Laptop
*Website interactives/ links/ / Geogebra Applets
- This is a resource file on 'vertically opposite angles'
It has been created and uploaded by Sucheta, Mathematics teacher, GHS Thyamangondlu
*Process:
- Reiterate that when a transversal intersects parallel lines, several pairs of congruent and supplementary angles are formed.
- Have students draw two parallel lines and a third line(transversal) intersecting those two lines on their own paper. Direct them to think about any angle relationships they see. Have them discuss their conjectures with a partner.
- The teacher can next project the GeoGebra worksheet and discuss about types of angles and their relationships with the class .
- Finally the teacher and students can summarize together the angle relationshipsalong with their characteristics.
Linear pair of angles - adjacent and supplementary
- Vertical angles - congruent
- Corresponding angles -congruent
- Alternate interior angles - congruent
- Same side interior angles - supplementary
- Alternate exterior angles - congruent
- Same side exterior angles - supplementary
*Developmental Questions :(What discussion questions)
- How many pairs of corresponding angles are there ?
- What is true about corresponding angles formed when parallel lines are cut by a transversal?
- Compare different pairs of alternate interior angles. What do you notice?
- <EGD and <AHF are alternate exterior angles. What is another pair of alternate exterior angles?
- Compare different pairs of same-side interior angles. What do you notice?
- Compare different pairs of same-side exterior angles. What do you notice?
*Evaluation:
- What are the characteristics of linear angles (adjacent and supplementary) ?
- What do you observe about the angle measures of the linear angles?
*Question Corner:
- What do adjacent , alternate, linear , corresponding and consecutive mean individually
- What are complementary angles?
- What are supplementary angles ?
- What does it mean if two angles are congruent?
- What is the complement of 65 degrees
- What is the supplement of 70 degrees?
- Compare angle relationships formed by parallel lines vs. angle relationships formed by non-parallel lines.
Activity No 2 Angles formed when a transversal intersects parallel lines
- Estimated Time : 90 minutes
- Materials/ Resources needed
Laptop, geogebra file, projector and pointer.
- Prerequisites/Instructions, if any
- The students should have prior knowledge of parallel lines , transversal, angles and types of angles formed when a pair of parallel lines are intersected by a transversal.
- They should know what the terms interior, exterior, adjacent, alternate, consecutive, congruent, linear and corresponding mean.
- Students should know the definition of complementary angles, supplementary angles, and congruent angles.
- Multimedia resources:
Laptop
- Website interactives/ links/ / Geogebra Applets
- Process
- Reiterate that when a transversal intersects parallel lines, several pairs of congruent and supplementary angles are formed.
- Have students draw two parallel lines and a third line(transversal) intersecting those two lines on their own paper. Direct them to think about any angle relationships they see. Have them discuss their conjectures with a partner.
- The teacher can next project the GeoGebra worksheet and discuss about types of angles and their relationships with the class .
- Finally the teacher and students can summarize together the angle relationships.
Linear pair of angles - adjacent and supplementary
Vertical angles - congruent
Corresponding angles -congruent
Alternate interior angles - congruent
Same side interior angles - supplementary
Alternate exterior angles - congruent
Same side exterior angles - supplementary
- Developmental Questions
- How many pairs of corresponding angles are there ?
- What is true about corresponding angles formed when parallel lines are cut by a transversal?
- Compare different pairs of alternate interior angles. What do you notice?
- <EGD and <AHF are alternate exterior angles. What is another pair of alternate exterior angles?
- Compare different pairs of same-side interior angles. What do you notice?
- Compare different pairs of same-side exterior angles. What do you notice?
Evaluation
- What are the characteristics of linear angles (adjacent and supplementary) ?
- What do you observe about the angle measures of the linear angles?
- Question Corner
- What do adjacent , alternate, linear , corresponding and consecutive mean individually
- What are complementary angles?
- What are supplementary angles ?
- What does it mean if two angles are congruent?
- What is the complement of 65 degrees
- What is the supplement of 70 degrees?
- Compare angle relationships formed by parallel lines vs. angle relationships formed by non-parallel lines.
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
- Process/ Developmental Questions
- Evaluation
- Question Corner
Hints for difficult problems
Project Ideas
Math Fun
Usage
Create a new page and type {{subst:Math-Content}} to use this template