Acceleration due to Gravity
| Philosophy of Science |
While creating a resource page, please click here for a resource creation checklist
Concept Map
Textbook
To add textbook links, please follow these instructions to: (Click to create the subpage)
Additional information
Useful websites
Reference Books
Teaching Outlines
Concept #
Learning objectives
Notes for teachers
These are short notes that the teacher wants to share about the concept, any locally relevant information, specific instructions on what kind of methodology used and common misconceptions/mistakes.
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ simulations
- Process (How to do the activity)
- Developmental Questions (What discussion questions)
- Evaluation (Questions for assessment of the child)
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ simulations
- Process (How to do the activity)
- Developmental Questions (What discussion questions)
- Evaluation (Questions for assessment of the child)
- Question Corner
Concept #
Learning objectives
Notes for teachers
These are short notes that the teacher wants to share about the concept, any locally relevant information, specific instructions on what kind of methodology used and common misconceptions/mistakes.
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ simulations
- Process (How to do the activity)
- Developmental Questions (What discussion questions)
- Evaluation (Questions for assessment of the child)
- Question Corner
Activity No #
- Estimated Time
- Materials/ Resources needed
- Prerequisites/Instructions, if any
- Multimedia resources
- Website interactives/ links/ simulations
- Process (How to do the activity)
- Developmental Questions (What discussion questions)
- Evaluation (Questions for assessment of the child)
- Question Corner
Project Ideas
Fun corner
Usage
Create a new page and type {{subst:Science-Content}} to use this template
Acceleration due to gravity
Concept flow
Some of the key ideas we will cover in this section are:
- Gravitational force due to the Earth produces an acceleration in the objects. This is the force acting on a freely falling object.
- The value of acceleration is not dependent on the mass.
- All freely falling bodies gain same acceleration.
Free fall and acceleration due to gravity
A freely falling body undergoes acceleration. This acceleration is caused by the gravitational force exerted by the larger mass of the Earth. This is referred to as acceleration due to gravity. The Earth also undergoes an acceleration due to the gravitational force exerted by the object. We do not notice it because of the mass of the Earth.
The acceleration that an object experiences because of gravity when it falls freely close to the surface of a massive body, such as a planet is also known as the acceleration of free fall, its value can be derived as follows.
Let M be the mass of the Earth and m be the mass of the object that is subjected to free fall. Let R be the radius of the Earth and h be the height above the surface of the Earth.
The gravitational force on the object due to the Earth is given by
Fg = G Mm (R + h) 2 = m a (by Newton's second law of motion)
If R >>h, then this can be written as
a = GM/ R2
We call this acceleration due to gravity and is indicated by “g”. Please note that this is very different from the Universal Gravitational Constant “G”.
Notice the important result here that the acceleration due to gravity is independent of the mass of the object.
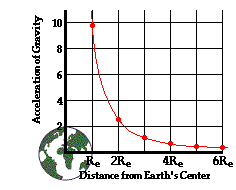
In the case of the Earth, g comes out to be approximately 9.8 m/s2, though the exact value depends on location because of two main factors: the Earth's rotation and the Earth's equatorial bulge.
An object that is allowed to fall freely will, if the effects of air resistance are ignored, gather speed (accelerate) at a rate of about 9.8 m/s2. If dropped from rest, it will have fallen 4.9 m and be traveling at a speed of 9.8 m/s after 1 second. After 2 seconds, it will have fallen a further 14.7 m and be traveling at 19.6 m/s. After 3 seconds, it will have fallen a further 24.5 m and be traveling at 29.4 m/s.
Variation of 'g' at various places on Earth
The value of “g” varies according to the effect of the Earth's rotation. If we have a mass hanging in equilibrium from a spring balance at the North Pole, there are two forces acting on the mass, Fg (= mg) and “w” which is the force with which the spring will pull on the mass. An equal and opposite force “w” acts on the spring downwards and this “w” will be read as the weight of the object.
Since the body is in equilibrim, there is no net force and there is no acceleration.
Therefore, mg – w = 0; w = mg.
However at the equator, the gravitational force is still Fg (= mg) and an equal and opppsite force w1 causes the spring to pull on the mass. An equal and opposite force “w1 ” acts on the spring downwards and this “w1 ” will be read as the weight of the object.
The object at the equator, has an acceleration, which is given by the centripetal acceleration.
mg - w1 = m v2/ R
where v is the rotational speed of the Earth and R is the radius.
W1 = m g' = m (g - v2/ R)
Difference in acceleration, g - g' = 0.0337 m/s2
Please see section on Additional Information for details of difference in “g”.
Variation due to the shape of the Earth
When the Earth was formed it was still molten. Due to the rotation, more mass moved towards the centre. This has resulted in the Earth being flatter at the poles and fatter at the equator. There is a difference of about 20 km in the distance from the centre at the equator and the poles. Therefore, an object closer to the equator will have a higher velocity and therefore, higher centripetal acceleration. This will result in a difference in the acceleration due to gravity. Nonfree fall
In the discussion above, we have assumed that an object is falling through vacuum – there are no other forces other than gravitational forces acting on it Although a feather and a coin will fall equally fast in a vacuum, they will fall differently in air. This is due to the presence of resistive forces.
Newton's laws apply both in vacuum and in the presence of air resistance. The important thing to remember is that acceleration is dependent on the net force.
If the Earth had no atmosphere, an object dropped from a great height would keep accelerating at a rate of 9.8 m/s2 until it hit the ground. For example, if a person fell from an aircraft at an altitude of 10,000 m, they would be travelling at about 442 m/s (1500 km/ hr) by the time they landed. In practice, this doesn't happen because of air resistance. The faster an object falls, the greater is the air resistance (called air drag) acting on it. Air drag depends on the surface area of the falling object and the speed.
At a certain velocity, known as the terminal velocity, the downward force of gravity is balanced out by the upward force of air resistance and there is no further acceleration. And it continues to move at the same terminal velocity till it reaches the ground.
The effect of air resistance
If there were no atmosphere, all objects would fall at the same rate. This happens, for example, on the Moon. In one of the most memorable moments of the space program, David Scott, commander of the Apollo 15 mission, standing on the Moon's surface, dropped two objects – a geological hammer and a falcon's feather (the Apollo 15 lunar module was called Falcon) – at the same time from the same height. The feather didn't drift down, meanderingly, as it would have done on Earth. Instead, in the airless vacuum of space, it fell straight, without a flutter, keeping pace with the hammer and reaching the lunar surface at the same instant.
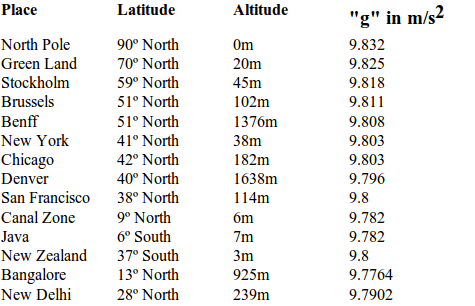
Variance of “g” on Earth
In the case of the Earth, g comes out to be approximately 9.8 m/s2 (32 ft/s2), though the exact value depends on location because of two main factors: the Earth's rotation and the Earth's equatorial bulge. We saw that the value of “g” depends on the mass of the Earth and the distance from the centre. At a distance of twice the radius of the Earth, the value of “g” drops to 2.45 m/s2.
The shape of the curve suggests that the value of “g” changes according to the inverse square law
Value of “g” at different places