Difference between revisions of "Slope of a line"
| Line 110: | Line 110: | ||
# Ask the students to inspect the Slope with the help of relation between X and Y | # Ask the students to inspect the Slope with the help of relation between X and Y | ||
# What is the slope of a horizontal and a Vertical line? | # What is the slope of a horizontal and a Vertical line? | ||
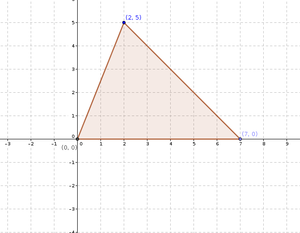
| + | # Find the slope of three segments of the triangle given below. | ||
| + | [[Image:Assessment question to find the slope.png|300px]] | ||
==Concept 2== | ==Concept 2== | ||
Revision as of 10:37, 13 August 2014
| Philosophy of Mathematics |
While creating a resource page, please click here for a resource creation checklist.
Concept Map
Textbook
Please click here for Karnataka and other text books.
Additional Information
Useful websites
Reference Books
Teaching Outlines
Concept 1
understanding what is a Slope
Learning objectives
- Slope is measure of the steepness of a line.
- Students will understands that slope increases with the steepness.
- Students will recognize and make a connection between the magnitude of the slope and the steepness of a line.
- Students will understands that Slope is a number (magnitude).
- Students understands that Slope is the orientation or inclination of a line with the X-axis.
- Students should also be able to draw a line with a specific slope.
Notes for teachers
Slope is a number which represents the steepness of a line Students can relate the slope of line to the tangent of the angle of elevation.
Activities
- Activity No1
understanding What is a slope?
Procedure
Ask the students to observe the given table describe the pattern using words .
Ask them to plot and join the points on grids and ask how the line segments is visible in the graph.
ask them to write the relation between X and Y.
(we can come to a conclusion that the larger the absolute value of the number,the linebecomes steeper.)
| X | Y |
| 6 | 1 |
| 7 | 2 |
| 8 | 3 |
| 9 | 4 |
| X | Y |
| 4 | 1 |
| 5 | 3 |
| 6 | 5 |
| X | Y |
| 6 | 1 |
| 7 | 4 |
| 8 | 7 |
Assessment Questions
- Write the relation between X and Y(as an equation)
- plot the other points following the same pattern and join the points
- How do we differentiate these lines from one another?from the inclination with the x-axis i.e bending of line towards x-axis
- Can we visualise the angle so formed by the line with the X-axis?
- What is the relation between the bending of line and angle formed by it with the X-axis?
We can conclude that bending(orientation of line)increases with the increse in the angle or we can say line becomes steeper with the angle of inclination.
The slope of a line is a number that measures its "steepness" It is the change in y for a unit change in x along the line.
- How do we measure the steepness of line?
- Ask the students to measure Slope of other lines
- Ask the students to inspect the Slope with the help of relation between X and Y
- What is the slope of a horizontal and a Vertical line?
- Find the slope of three segments of the triangle given below.
Concept 2
Positive and Negetive Slope
Learning objectives
- Students should also be able to visualise a line with positive and negetive Slope
- Students should also be able to differentiate a line with positive and negetive Slope
Notes for teachers
If the change in Y with the value of X decreases corresponding line will have Negetive Slope If the change in Y with the value of X increases corresponding line will have Positive Slope
Activities
- Activity No #1
The following Geogebra applet helps in visualising Positive and negetive Slope
Geogebra Applet
Concept 3
From the following geogebra applet we can visualise that slope of two parallel lines are same and slope of two perpendicular lise are negetive resiprocals of each other
Slopes of Parallel and Perpendicular lines