Circles Tangents Problems

Problem 1

Tangents AP and AQ are drawn to circle with centre O, from an external point A. Prove that ∠PAQ=2.∠ OPQ

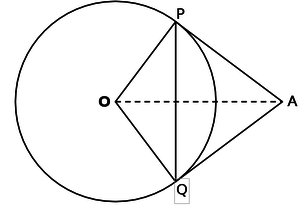
Interpretation of the problem

1. O is the centre of the circle and tangents AP and AQ are drawn from an external point A.
2. OP and OQ are the radii.
3. The students have to prove thne angle PAQ=twise the angle OPQ.

Concepts used

1. The radii of a circle are equal.
2. In any circle the radius drawn at the point of contact is perpendicular to the tangent.
3. The tangent drawn from an external point to a circle a] are equal b] subtend equal angle at the centre c] are equally inclined to the line joining the centre and extrnal point.
4. Properties of isoscles triangle.
5. Properties of quadrillateral ( sum of all angles) is 360 degrees
6. Sum of three angles of triangle is 180 degrees.

Algorithm

OP=OQ ---- radii of the same circle OA is joined.
In quadrillateral APOQ ,
∠APO=∠AQO=$90^{0}$ [radius drawn at the point of contact is perpendicular to the tangent]
∠PAQ+∠POQ=$180^{0}$
Or, ∠PAQ+∠POQ=$180^{0}$
∠PAQ = $180^{0}$-∠POQ ----------1
Triangle POQ is isoscles. Therefore ∠OPQ=∠OQP
∠POQ+∠OPQ+∠OQP=$180^{0}$
Or ∠POQ+2∠OPQ=$180^{0}$
2∠OPQ=$180^{0}$- ∠POQ ------2
From 1 and 2
∠PAQ=2∠OPQ

Problem-2

In the figure two circles touch each other externally at P. AB is a direct common tangent to these circles. Prove that
a). Tangent at P bisects AB at Q
b). ∠APB=90° (Exescise-15.2, B.3)

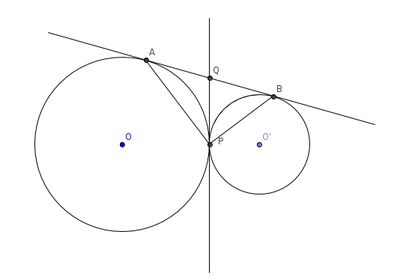
Interpretation of the problem

1. In the given figure two circles touch externally.
2. AB is the direct common tangent to these circles.
3. PQ is the transverse common tangent drawn to these circles at point P.
4. Using the tangent properties students have to show AQ=BQ and ∠APB=90°

Concepts used

1. The tangent drawn from an external point to a circle
   a) are equal
   b] subtend equal angle at the center
   c] are equally inclined to the line joining the center and external point.
2. Angle subtended by equal sides are equal.
3. Axiom-1:- "Things which are equal to same thing are equal"
   

[Click here for geogebra animation]

Algorithm

In the above figure AB is direct common tangent to two circles and PQ is the Transverse common tangent.
'Step-1'Bold text
AQ=QP and BQ=QP (Tangents drawn from external point are equal)
By axiom-1, AQ=BQ
∴tangent at P bisects AB at Q.

problem 3 [Ex-15.2 B.7]

Circles $C_{1}$ and $C_{2}$ touch internally at a point A and AB is a chord of the circle$C_{1}$ intersecting $C_{2}$ at P, Prove that AP= PB.

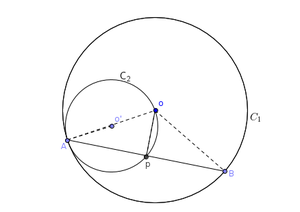
Concepts used

1. The radii of a circle are equal
2. Properties of isosceles triangle.
3. SAS postulate
4. Properties of congruent triangles.

Prerequisite knowledge

1. The radii of a circle are equal.
2. In an isosceles triangle angles opposite to equal sides are equal.
3. All the elements of congruent triangles are equal.

Algoritham

In ∆AOB
AO=BO [Radii of a same circle]
∴ ∠OAB = ∠OBA --------------I [∆AOB is an isosceles ∆}
Then,
In ∆AOP and ∆BOP,
AO = BO [Radii of a same circle]
OP=OP [common side]
∠OAP = ∠OBP [ from I]
∴ ∆AOP ≅ ∆BOP [SAS postulate]
∴ AP = BP [corresponding sides of congruent triangles ]

problem-4

In the given Quadrilateral ABCD , BC=38cm , QB=27cm , DC=25cm and AD⊥DC find the radius of the circle.(Ex:15.2. A-6)