Difference between revisions of "A Kite and its properties"
Jump to navigation
Jump to search
m (added Category:Quadrilaterals using HotCat) |
|||
| (5 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
| − | + | = A Kite and its properties = | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
===Learning objectives=== | ===Learning objectives=== | ||
# A kite is a quadrilateral with two distinct pairs of adjacent sides that are congruent. | # A kite is a quadrilateral with two distinct pairs of adjacent sides that are congruent. | ||
| Line 57: | Line 23: | ||
{| style="height:10px; float:right; align:center;" | {| style="height:10px; float:right; align:center;" | ||
|<div style="width:150px;border:none; border-radius:10px;box-shadow: 5px 5px 5px #888888; background:#f5f5f5; vertical-align:top; text-align:center; padding:5px;"> | |<div style="width:150px;border:none; border-radius:10px;box-shadow: 5px 5px 5px #888888; background:#f5f5f5; vertical-align:top; text-align:center; padding:5px;"> | ||
| − | ''[http:// | + | ''[http://karnatakaeducation.org.in/?q=node/305 Click to Comment]''</div> |
|} | |} | ||
*Estimated Time: 30 minutes. | *Estimated Time: 30 minutes. | ||
| Line 85: | Line 51: | ||
# Compare kite with other quadrilaterals and make a list of similarities and differences between them. | # Compare kite with other quadrilaterals and make a list of similarities and differences between them. | ||
| − | + | [[Category:Quadrilaterals]] | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
Latest revision as of 13:55, 5 August 2020
A Kite and its properties
Learning objectives
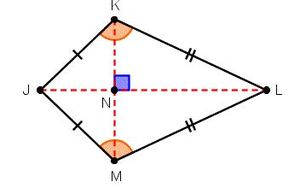
- A kite is a quadrilateral with two distinct pairs of adjacent sides that are congruent.
- The two pairs of congruent sides meet at two different points.
- A kite can also be described as a quadrilateral with an axis of symmetry along one of its diagonals.
- Kites have a couple of properties that will help us identify them from other quadrilaterals.
- The diagonals of a kite meet at a right angle.
- Kites have exactly one pair of opposite angles that are congruent.
- Diagnols intersect at right angles.
<K = <M. This is the only pair of congruent angles because <J and <L have different measures.
Notes for teachers
Source : http://www.ask.com/question/what-is-a-kite-in-geometry
Summary :
- A kite is sometimes also known as a deltoid.
- A kite, may be either convex or concave, but the word "kite" is often restricted to the convex variety. A concave kite is sometimes called a "dart" or "arrowhead".
Activity No # 1. Paper kite
- Estimated Time: 30 minutes.
- Materials/ Resources needed :
- A4 sheet of paper.
- Prerequisites/Instructions, if any
- Neat paper folding skills.
- Ability to follow instructions.
- Multimedia resources
- Website interactives/ links/ / Geogebra Applets
This activity has been taken from the website : http://www.transum.org/software/Fun_Maths/kite/
- Process:
- Fold an A4 sheet of paper as shown in the figures to make a kite.
- Developmental Questions:
- Which is the figure formed ?
- What is special about this quadrilateral ?
- How many sides does a kite have ?
- Are all sides equal ?
- Mark the diagnols ? What do you notice about them ?
- Evaluation:
- Were the students able dto recognise the properties of a kite.
- Question Corner
- Compare kite with other quadrilaterals and make a list of similarities and differences between them.